What Is The Probability That Only The Receiver Needs Service During The Warranty Period?

Consider purchasing organisation of audio components consisting of receiver; pair Of speakers, and CD player: Let A exist the event that the receiver functions properly throughout the marranty the flow, Az be the event that the speakers function properly throughout the warranty flow, and Az be result that the CD actor functions properly throughout the warranty period _ Suppose that these events are (mutually) independent with P(Ai) 0.91, P(A2) 0.97 and PAz) 0.seventy (Round vour answers to iv decimal pl

Consider purchasing system of audio components consisting of receiver; pair Of speakers, and CD player: Allow A be the event that the receiver functions properly throughout the marranty the menstruum, Az be the event that the speakers function properly throughout the warranty period, and Az be outcome that the CD thespian functions properly throughout the warranty catamenia _ Suppose that these events are (mutually) contained with P(Ai) 0.91, P(A2) 0.97 and PAz) 0.70 (Circular vour answers to four decimal places.) What the probability that all 3 components function properly throughout the warranty menstruum? What the probability that at least one component needs service during the warranty period? What the probability that all iii components need service during the warranty menstruation? What is the probability that merely the receiver needs service during the warranty menses? What the probability that exactly one of the iii components needs service during the warranty period?

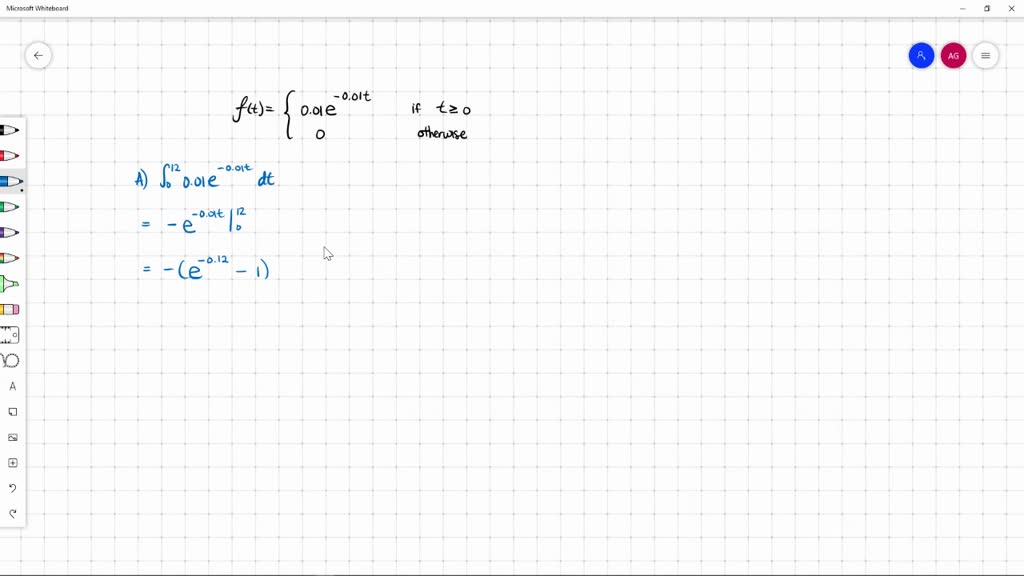
Unless stated to the contrary, compute all monetary answers to the nearest dollar: A manufacturer guarantees a product for ane year. The fourth dimension to failure of the production after information technology is sold is given by the probability density part $$ f(t)=\left\{\begin{array}{ll} .01 e^{-.01 t} & \text { if } t \geq 0 \\ 0 & \text { otherwise } \stop{assortment}\correct. $$ where $t$ is time in months. What is the probability that a buyer chosen at random volition have a product failure (A) During the warranty menstruum? (B) During the second twelvemonth after purchase?

In this problem, it is given that a manufacturer of a consumer electronics product, expects 2% of units to neglect during the warranty menstruum. A sample of 500 independent units is tracked for warranty operation. Let X denote the number of failures during the warranty period. So X is a binomial random variable with N is equal to 500 And P is two%. That is 0.02. We know that if X is a binomial random variable, so probability of X equal to Ten is n. C. X into periods 2, X into 1 minus P. Res two and minus 10. Nosotros're x ranges from 01 up to N. And then this is equal to Here, North is 500, And so 500 c. x. P is 0.02 In two, 0.02 raised to eggs In 2, 1 -0.02. That is 0.98, Raised to 500 minus 10. In the start part it is asked what is the probability that night phase during the warranty period? And then we have to detect probability of 10 equal to zero, Probability of x equal to zero is equal to 500 C zero here. X cipher. So 500 C aught Into 0.02, raised to zero Into 0.98 Raised to 500 0. That is 0.98 raised to 500. This is equal to 500, c is one, 0.0, tourists to zero is again one, so one in 21 into zero betoken 98 raised to 500. This is equal to four betoken ane's hawkeye. 24 into 10 raised to minus faith. This tin can likewise be written anus zero point 00 00 41. So the probability that none fails during the warranty menstruum is iv.1024 into x days to -5 Which is equal to 0.000041. In the side by side part. It is asked what is the expected number of failures during the warranty period? That is we have to observe expected eggs. We know that for the binomial random variable eggs expected X is np. So this is equal to Our end -500 Into slice 0.02. So 500 into 0.02 Which is equal to x. So The expected number of failures during the warranty period is 10. In the terminal function it is asked what is the probability that more than than two units failed during the warranty period? That is we have to find probability of X greater than two. This is equal to 1. My nurse probably be off X Less than or equal to ii. This is equal to one. My nurse Probability of ten equal to nix as the binomial random variable 10. Starts from zero. We will get-go calculating probabilities from X equal to nix. The probability of x equal to nix plus probability of X equal to well Plus probability of ten equal to two one. Miner's probability of Ten equal to zero. Because probability of 10 equal to i Plus probability of x equal to two. This is equal to just my nurse first we will find for X equal to zero. Then this is 500 C zero Into 0.02 raised to zero into naught point 98. Raised to 500 0. That is 500. This is a probability of 10 equal to zero. Now we will discover probability of five is equal to 1. So it is 500 C1 In two. 0.02 raised to one In 2 0.98 Raised to 500 -1. That is 499 plus. Now we will find for X equal to ii. So this is 500 C two In 2 0.02 there's ii Into 0.98 Raise to 500 -ii. That is four 98. This is a quite as well. But my nurse four point 1024 into ten degrees to minus v. This is probability of Ten equal to zero four.102. 4 into 10 days to -5 plus four indicate i ten half dozen. one Into 10 years. to -4 This is probability of 10 equal to one plus two signal 1315 In. To tenders to -3. This is a probability of x equal to two. Then now nosotros have to simplify this. This is equal to what my nurse zero bespeak 04 1024 Into 10 days. To my nursery. But we will take 10 years to -3 common from both the towns. So for the time beingness we will write only 0.410 to iv plus zero point 41 861 into ten days to -three. We will write it exterior plus 2.1315 Into ten days to -3. So we had taken into lawn tennis to -3 comin from all the iii tubs In 2, x years to minus iii. So now we volition simplify this. This is equal to 1. My nurse two points 591 134 Into 10 ways to -3. Now nosotros will multiply this by ten degrees to minus three. And so nosotros volition become this is equal to i minus zero bespeak 00 259 11 34. And then finally nosotros become this is equal to naught 99 74, 0.9974. Then the probability that more than than two units neglect during the warranty period is 0.9974.

In this problem, information technology is given that a manufacturer of a consumer electronics production, expects 2% of units to fail during the warranty period. A sample of 500 independent units is tracked for warranty operation. Let X announce the number of failures during the warranty period. So X is a binomial random variable with Northward is equal to 500 And P is two%. That is 0.02. We know that if X is a binomial random variable, then probability of X equal to Ten is n. C. Ten into periods ii, X into one minus P. Res two and minus 10. We're x ranges from 01 upward to N. So this is equal to Here, N is 500, So 500 c. x. P is 0.02 In 2, 0.02 raised to eggs In two, 1 -0.02. That is 0.98, Raised to 500 minus X. In the first part information technology is asked what is the probability that night phase during the warranty period? So we have to find probability of X equal to zero, Probability of 10 equal to zero is equal to 500 C aught here. X zero. Then 500 C nix Into 0.02, raised to zero Into 0.98 Raised to 500 0. That is 0.98 raised to 500. This is equal to 500, c is one, 0.0, tourists to zero is again one, and then ane in 21 into zero point 98 raised to 500. This is equal to four indicate i'south hawkeye. 24 into 10 raised to minus religion. This can also be written anus aught point 00 00 41. So the probability that none fails during the warranty period is 4.1024 into ten days to -5 Which is equal to 0.000041. In the next part. It is asked what is the expected number of failures during the warranty period? That is we take to discover expected eggs. Nosotros know that for the binomial random variable eggs expected 10 is np. So this is equal to Our end -500 Into piece 0.02. So 500 into 0.02 Which is equal to x. And so The expected number of failures during the warranty period is 10. In the last role it is asked what is the probability that more than two units failed during the warranty flow? That is nosotros have to find probability of X greater than ii. This is equal to i. My nurse probably be off X Less than or equal to two. This is equal to one. My nurse Probability of x equal to zippo as the binomial random variable X. Starts from zip. We will beginning calculating probabilities from X equal to cypher. The probability of x equal to naught plus probability of X equal to well Plus probability of ten equal to two ane. Miner'south probability of X equal to cypher. Because probability of X equal to one Plus probability of ten equal to ii. This is equal to only my nurse outset we will discover for X equal to nada. Then this is 500 C zero Into 0.02 raised to zero into zero point 98. Raised to 500 0. That is 500. This is a probability of 10 equal to null. Now nosotros will find probability of 5 is equal to one. Then information technology is 500 C1 In ii. 0.02 raised to one In ii 0.98 Raised to 500 -one. That is 499 plus. Now we will find for X equal to ii. So this is 500 C ii In ii 0.02 at that place's two Into 0.98 Raise to 500 -2. That is 4 98. This is a quite too. But my nurse four betoken 1024 into ten degrees to minus five. This is probability of X equal to zilch iv.102. four into 10 days to -5 plus four point i ten half dozen. 1 Into ten years. to -4 This is probability of x equal to one plus two signal 1315 In. To tenders to -three. This is a probability of ten equal to ii. So now nosotros take to simplify this. This is equal to what my nurse zero indicate 04 1024 Into 10 days. To my nursery. But nosotros will take 10 years to -3 common from both the towns. So for the time being we will write only 0.410 to iv plus zero point 41 861 into ten days to -3. Nosotros will write it outside plus 2.1315 Into x days to -iii. So we had taken into tennis to -3 comin from all the 3 tubs In ii, 10 years to minus three. So now we will simplify this. This is equal to one. My nurse two points 591 134 Into 10 ways to -3. At present we will multiply this past 10 degrees to minus iii. And then nosotros will get this is equal to 1 minus zero point 00 259 11 34. And so finally we become this is equal to zero 99 74, 0.9974. And then the probability that more than two units fail during the warranty flow is 0.9974.

We're told that a manufacturer guarantees a product for one year and the time to failure of the product later his soul is given past this probability density function. So we have kind of an exponentially decomposable thing. And and so plainly for T. Lesson null, information technology'south just there's no zero probability. That's then information technology'south only really divers for greater than time greater than zilch. So they inquire us um what is the probability that a buyer chosen at random will have a product failure in In during the warranty period? So the warranty period is one twelvemonth. And we got to be careful because they said in here T. Is in months. So we tin get from 0 to 12 months And we tin can integrate this. And we did that evaluate presents around 12 when we go 11.3%,, So it'south eleven.iii% risk that some person volition have their production neglect in one year. Or if you have a grouping of people, you know, just 11 point if you have 100 of them, 11.3 of them will xi of them will take their product fail roughly? 11 will have their product failing one after ane twelvemonth. It seems like a lot. And so anyway, I'm non certain how reasonable this is, but that seems like a lot of product values i in x. Uh and so then they ask usa how, during the 2nd year after purchase, so how many will then neglect after, you know, it's a second during the 2nd year. And that ways going from integrating from naught from 12 to 24, That winds upward beingness 10%. So after After two years, let's see here, nosotros'll have well-nigh 20% of them neglect, so lxxx% of them will notwithstanding be going And then after they ask us um trouble 19, What is the probability that the production will final at least one yr? Well the probability that at last is one minus the probability that failed in 1 year. Then it failed 11.11.vii eleven.3% of the time it failed in one yr. So that ways that the residual of them lasted. And so that means 88.7% of them made it to i yr. And so once again, because they say they requite united states of america the hint that the integral the integral of this thing Over from 0 to Infinity is one. So we know that we accept that You lot know, the total is i Or 100%. So the probability that they 13, of them failed afterwards one year. That means 88.7% of them lasted one year. Yes.

The random railroader is normally distributed with the main 22 and a standard divergence of four. We want to compute the probability that this random variable is less than 12. Since one twelvemonth has 12 months, we can transform it into a standard normal distribution that has. We subtract the chief and divided by the Standard Education, so it echoes the probability that A standard normal random variable is less than -2.v and use the table in appendix. C. This probability has a point 00 62 All you can rewrite it as .62%.

What Is The Probability That Only The Receiver Needs Service During The Warranty Period?,

Source: https://itprospt.com/num/6940440/consider-purchasing-system-of-audio-components-consisting

Posted by: simpsonprinnexparm.blogspot.com

Share this post